Exploring Anderson Localization of Light
by By Dominic Siriani
Over 50 years ago, P. W. Anderson first suggested that the diffusion of electrons can be frozen in a sufficiently disordered lattice. The backbone of the idea is in the quantum mechanical model of electron transport, in which the particles are also waves. As an intuitive explanation, one can consider the sum of the probability amplitudes of different paths the electron can take. The phases of these amplitudes must be taken into account, and as with all waves, constructive or destructive interference can occur. For a medium with strongly scattering disordered defects, the result of such interference can lead to the localization of the electron wavefunction in one place (
i.e., diffusion is inhibited).
As a wave phenomenon, Anderson localization can occur to any wave, be it quantum waves, acoustic waves, or, as is the topic of this post, electromagnetic waves. Observing Anderson localization with light is actually one of the less complex manifestations of the effect, since, unlike electrons, photons do not interact with one another. And this sort of phenomenon could have some really neat applications.
At this year’s Frontiers in Optics meeting, Prof. Arash Mafi will be giving an invited talk on transverse Anderson localization in optical fibers. I took a look at some of Prof. Mafi’s recent work to see what their cutting edge research has realized. What they’ve been able to demonstrate is essentially a “coreless” fiber that provides waveguiding, all thanks to localization in a disordered medium.
Prof. Mafi’s group created a disordered optical fiber by randomly mixing strands of polystyrene (PS) and polymethyl methacrylate (PMMA) and drawing it into a square fiber (see figure). The result is an optical fiber with random regions of refractive indices of 1.59 (PS) and 1.49 (PMMA) in the transverse directions, and little to no index variation along the length of the fiber. What this allows for is the realization of two-dimensional Anderson localization. Or in more plain terms, the beam can travel down the fiber without expanding in the transverse directly, a feat usually accomplished with a step-index waveguide, which operates on very different physical principles.
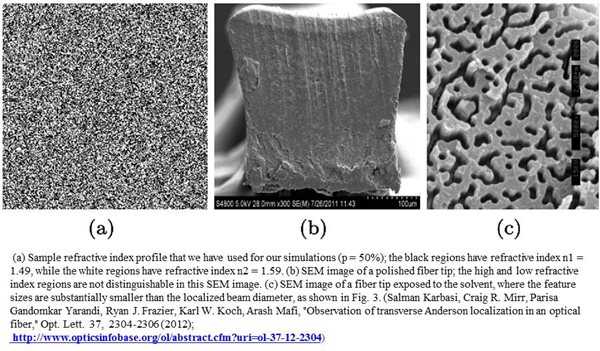 http://www.opticsinfobase.org/ol/abstract.cfm?uri=ol-37-12-2304)
http://www.opticsinfobase.org/ol/abstract.cfm?uri=ol-37-12-2304)
Using this fiber, the research group was able to demonstrate propagation of a beam down a fiber of length 60 cm. After propagating down the first 2 cm of the fiber, the beam expands to its full size, on average about 31 um in radius (see figure). However, that’s as far as the localization effect will allow the beam to “diffuse,” and that beam width is maintained as the light continues to travel down the fiber. This is an excellent example of the localization effect on the fundamental level, and in the practical sense it gives a good idea of the potential for exploiting localization in actual devices.

Disclaimer: Opinions, interpretations, conclusions, and recommendations are those of the author and are not necessarily endorsed by the United States Government and MIT Lincoln Laboratory.
Posted: 4 Sep 2013 by By Dominic Siriani